The Potts model is an obvious generalization of the Ising model to an arbitrary number, Q, of discrete states. Proposed by Domb to his grad student, Potts.
As for Ising model, if J > 0, the energy is lower if neighboring spins are the same (aligned).
2-state Potts 
Ising.
Q>2 model has different critical exponents, interesting phase structure.
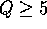has first order transition in two dimensions.