


Next: Improvement of Poor
Up: No Title
Previous: Clarification of Eigenvalue
- So Poor Person's Conjugate Gradient is very good at large
eigenvalues, but converges slowly for small eigenvalues of A.
- Note A is positive definite for simple pde's
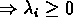 .
.
- What we would like to do is to arrange first that
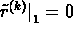 , then we see that equations give
, then we see that equations give 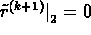 , and then the next iteration will give
, and then the next iteration will give 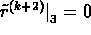 , and so on.
, and so on.
- So qualitative goal of real conjugate gradient is to successively
minimize along the ``principal directions of matrix A.''
- As described in my optimization notes, this is easily understood
in case n=2 in terms moving in hills and valleys.
Geoffrey Fox, Northeast Parallel Architectures Center at Syracuse University, gcf@npac.syr.edu
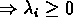 .
.
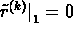 , then we see that equations give
, then we see that equations give 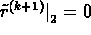 , and then the next iteration will give
, and then the next iteration will give 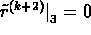 , and so on.
, and so on.
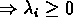 .
.
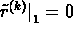 , then we see that equations give
, then we see that equations give 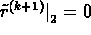 , and then the next iteration will give
, and then the next iteration will give 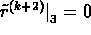 , and so on.
, and so on.