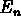 whose probability of occupation varies with t.
whose probability of occupation varies with t.
 is ``we have observed n events.''
is ``we have observed n events.''
 Note only one
Note only one 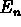 occupied at a time
occupied at a time
 and
and 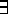 chance
chance 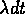 of a transition
of a transition 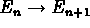 .
.
 This is a pure birth process.
This is a pure birth process.
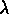 to
to 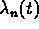 : a function of n
and t.
: a function of n
and t.
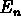 to
to  with chance
with chance 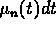 . So:
. So:
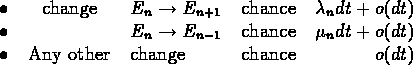
 : the probability of system being in state
: the probability of system being in state 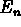 .
.
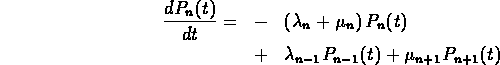
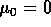 and
and 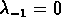 so that above is still
correct with n=0.
so that above is still
correct with n=0.