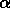Next:
Enter Mr. Bayes
Up:
3: Estimation of
Previous:
Maximum Likelihood Principle
Enter Mr. Bayes for Conditional Probablities---I
Now what we really want is the probability distribution of an estimate
of
.
We can formally get this by use of Bayes law:
First extend
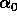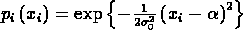to be defined for any value of
; and not just for
true value
.
What does this mean, as only one true
?
Introduce:
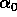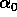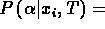Probability that true length is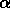
given measurements
and prejudice
T
.
Then by Bayes---or rather a slight extension of it,
which only differs from the ordinary Bayes Law by extra conditional
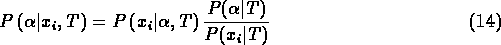
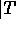in each probability.
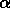Geoffrey Fox
,
Northeast Parallel Architectures Center
at Syracuse University,
gcf@npac.syr.edu
of 
.
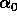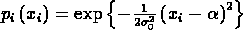to
be defined for any value of 
; and not just for 
true
value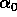 
.
?
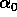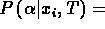Probability
that true length is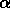 
given measurements 
and prejudice T.
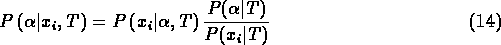
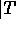in each probability.